The inductive fields are governed by the Maxwell equations solved in terms of electrodynamic potentials, i.e. the electric field and the magnetic field are expressed in terms of the scalar potential \(\Phi\) and the vector potential \( \vec{A} \). Inductive discharges usually have a cylindrically axisymmetric configuration where the fields are excited by an azimuthal driving current in a coil outside the plasma. In cylindrically symmetric systems the fields are described by the azimuthal component \(A_\phi\) of the vector potential.
PLASIMO offers two different approaches for solving the ICP fields: in frequency domain and in time domain.
ICP field calculation in frequency domain
The vector potential is calculated with boundary-relaxation technique, in which \( A_\phi \) in the plasma region is updated iteratively until the interior values are consistent with the boundary values, taking into account the additional contribution of the load coil. An advantage of this method is that there is no need to incorporate current-free regions in the computational domain.
This module assumes that a harmonic excitation current is present with an angular frequency \(\omega\). Furthermore, it assumes that the plasma frequencies are so high that the resulting fields cannot give rise to harmonic oscillations of the space charge. In this case only static space charges are present, for example those which are the result of ambipolar diffusion due to plasma inhomogeneities. The free currents are only due to conduction, for which Ohm's law is supposed to hold.
\begin{equation} \vec{J}_\Omega = \sigma \vec{E} = -i \omega \sigma \vec{A} \end{equation} \begin{equation} \nabla \times \nabla \times A_\phi = -(i \omega \mu \sigma + \omega^2 \mu \epsilon) A_\phi \end{equation}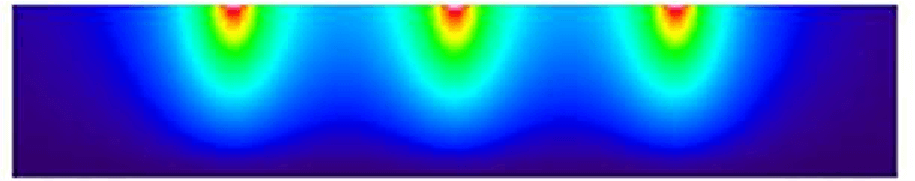
Figure 1: Calculation of the electromagnetic field in a cylindrical conducting region inside a co-axial load coil with three windings.
More information about this module can be found in [1] and [2].
ICP field calculation in time domain
In case of low pressure plasmas and low collision frequency, or at low field frequency, the electrons tend to travel important distances during the field period. This modules takes into account the plasma current, i.e. does not assume Ohm's law and resolves the wave in time. In this case the total current density \(J_t \) has two contributions: the driving current density exciting the electromagnetic waves and the plasma current density.
\begin{equation} J_t = J_{coil} + q_e n_e u_e, \end{equation}where \(q_e\) is the electron charge, \(n_e\) the electron density and \(u_e \) the electron velocity.
Using that for the azimuthal component \(E_\phi = - \frac{\partial A_\phi}{\partial t } \), the equation that is solved is:
\begin{equation} \nabla \times \nabla \times E_\phi + \mu \epsilon \frac{\partial^2 E_\phi}{\partial^2 t } = -\mu \left( \frac{\partial J_{coil,\phi}}{\partial t} + \frac{\partial (q_e n_e u_{e,\phi})}{\partial t } \right) \end{equation}Screen capture of a time-dependent ICP field simulation.
References
[1] Jan van Dijk. Modelling of Plasma Light Sources — an object-oriented approach. PhD thesis, Eindhoven University of Technology, The Netherlands, 2001.
[2] Jan van Dijk, Marc van der Velden, and Joost van der Mullen. A multi-domain boundary-relaxation technique for the calculation of the electromagnetic field in ferrite-core inductive plasmas. J. Phys. D: Appl. Phys., 35, 2748–2759, 2002.